Question

Find the equation of an ellipse whose axes lie along coordinates axes and which passes through (4, 3) and (- 1, 4).

Answer 1

Given that we need to find the equation of the ellipse passing through the points (4, 3) and (- 1, 4).

Let us assume the equation of the ellipse as

Substituting the point (4, 3) in (1) we get

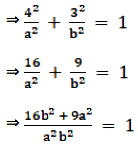
⇒ 16b² + 9a² = a² b² ..... - - (2)

Substituting the point (- 1,4) in (1) we get

⇒ b² + 16a² = a²b² ..... - - (3)

(3) × 16 - (2)

⇒ (16b² + 256a²) - (9a² + 16b²) = (16a²b² - a²b²)

⇒ 247a² = 15a²b² ⇒ 15b² = 247

⇒ b² = \(\cfrac{247}{15}\)

From (3)

The equation of the ellipse is

⇒ 7x² + 15y² = 247

∴ The equation of the ellipse is 7x² + 15y² = 247.