Derive an expression for equivalent capacitance of three capacitors when connected in series

Question

(i) Derive an expression for equivalent capacitance of three capacitors when connected in series

(ii) Derive an expression for equivalent capacitance of three capacitors when connected in parallel.

Answer 1

In fig. (a) Three capacitors of capacitances C₁, C₂, C₃ are connected in series between points A and D.

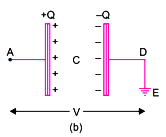
In series’ first plate of each capacitor has charge +Q and second plate of each capacitor has charge –Q i.e., charge on each capacitor is Q.

Let the potential differences across the capacitors C₁, C₂, C₃ be V₁, V₂, V₃ respectively. As the second plate of first capacitor C₁ and first plate of second capacitor C₂ are connected together, their potentials are equal. Let this common potential be VB.

Similarly the common potential of second plate of C₂ and first plate of C₃ is VC. The second plate of capacitor C₃ is connected to earth, therefore its potential VD=0. As charge flows from higher potential to lower potential, therefore

V_A>V_B>V_C>V_D.

Adding (i), (ii) and (iii), we get

If V be the potential difference between A and D, then 

V_A - V_D = V

\(\therefore\) From (iv), we get

If in place of all the three capacitors, only one capacitor is placed between A and D such that on giving it charge Q, the potential difference between its plates become V, then it will be called equivalent capacitor. If its capacitance is C, then

V = \(\cfrac QC\).....(vi)

Comparing (v) and (vi), we get