Question

A plane mirrorr is placed `22.5cm` in front of a concave mirrorr of focal length `10cm`. Find where an object can be placed between the two mirrorrs, so that the first image in both the mirrorrs coincides.
A. `20 cm` from concave mirrorr
B. `15 cm` from the concave mirrorr
C. `5 cm` from plane mirrorr
D. `7.5 cm` from plane mirrorr

Answer 1

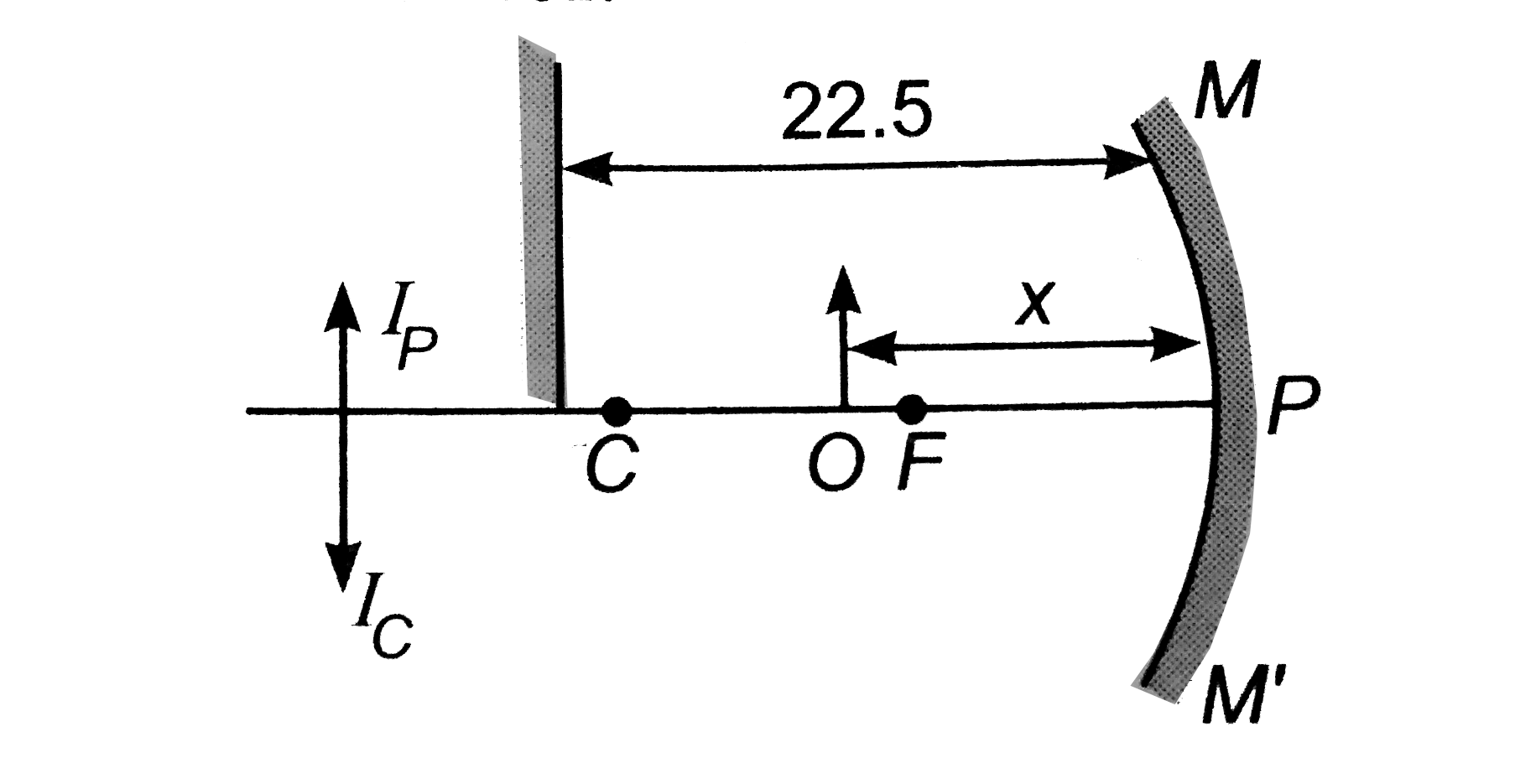
Correct Answer - B
As shown in figure, if the object is placed at a distance `x` from the concave mirrorr, its distance from the plane mirrorr will be `(22.5-x)`. So plane mirrorr will from equal and erect image of object at a distance `(22.5-x)` behind the mirrorr.
Now as according to given problem the image formed by concave mirrorr coincides with the image formed by plane mirrorr, therefore for concave mirrorr
`v=-[22.5+(22.5-x)]=-(45-x)`
And `u-x,` `(1)/(-(45-x^(2)))+(1)/(-x)=(1)/(-10)`
`(45)/((45x-x^(2)))=(1)/(10),` `x^(2)-45x+450=0`
`(x-30)(x-15)=0` `x=30` or, `x=15cm`
But as the distance between two mirrorrs is `22.5cm, x=30cm` is note admissible. So the object must be at a distance of `15 cm` from the concave mirrorr.