Question

Loads P₁ and P₂ are connected by a string passing over a fixed pulley. The centres of gravity of the loads are initially at the same height. Find the acceleration and the direction of the vertical motion of the centre of gravity of the system of loads if P₁ > P₂. 

Answer 1

Answer:

The centre of gravity moves downward with an acceleration 

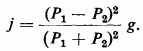
Explanation:

 During the time t each load is displaced from its initial position by a distance S = 1/2(at²) where

 Obviously, the centre of gravity of the system should then be displaced a distance L from the initial position, downward in the direction of the larger load (Fig.). According to the definition

of the centre of gravity, the distances of the centre of gravity to the loads should be inversely proportional to the size of these loads, i.e.,

Comparing the above result with the formula for the displacement in the case of uniform acceleration and substituting the value of the acceleration of the loads, we find that the centre of gravity should move downward with an acceleration

The acceleration of the centre of gravity is smaller than the acceleration of each of the loads separately.