Question

Prove that the current density of a metallic conductor is directly proportional to the drift speed of electrons.

Answer 1

Consider a uniform metallic wire XY of length l and cross sectional area A. A potential difference V is applied across the ends X andY of the wire. This causes an electric field at each point of the wire of strength

E = V/l. .....(1)

Due to this electric field, the electrons gain a drift velocity v_d  opposite to direction of electric field. If q be the charge passing through the cross-section of wire in t seconds, then current in wire I = q/t  ......(2)

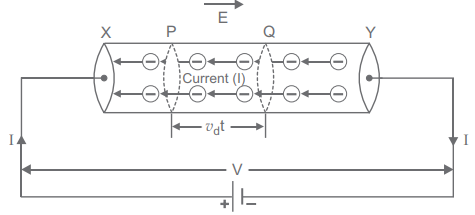
The distance traversed by each electron in time t

= average velocity x time =v_dt

If we consider two planes P and Q at a distance v_d t in a conductor, then the total charge flowing in time t will be equal to the total charge on the electrons present within the cylinder PQ. 

The volume of this cylinder = cross-sectional area x height = Av_dt

If n is the number of free electrons in the wire per unit volume, then the number of free electrons in the cylinder = n(Av_dt) If charge on each electron is - e(e = 1.6 x 10^-19C), then the total charge flowing through a cross-section of the wire

q = (nAv_dt)(-e) = - neAv_dt ......(3)

∴ Current flowing in the wire,

I =q/t = - (neAv_dt)/t

i.e., current I = -neAv_d .......(4)

This is the relation between electric current and drift velocity. Negative sign shows that the direction of current is opposite to the drift velocity