Question

The roots z₁, z₂, z₃ of the equation x³ + 3ax² + 3bx + c = 0, in which a, b, c are complex numbers, corresponding to the points A, B, C on the Gaussian plane. Find the centroid of the triangle ABC and show that it will be equilateral if a² = b.

Answer 1

Since z₁, z₂, z₃ are the roots of x³ + 3ax² + 3bx + c = 0.

We have

z₁ + z₂ + z₃ = -3a

⇒ z₁ + z₂ + z₃/3 = -a

and 

z₁z₂ + z₂ z₃ + z₃z₁ = 3a

Hence, the centroid of the triangle ABC is the point with affix −a. Now, the triangle will be equilateral if

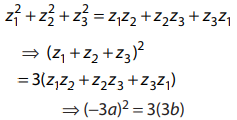
Therefore, the condition is a² = b.