Question

Let P be a point in the first octant, whose image Q in the plane x + y = 3 (that is, the line segment PQ is perpendicular to the plane x + y = 3 and the mid-point of PQ lies in the plane x + y = 3) lies on the z-axis. Let the distance of P from the x-axis be 5. If R is the image of P in the xy-plane, then the length of PR is.....

Answer 1

Given: P is a point in first octant Q is image of P in plane x + y = 3 lies on z-axis midpoint of PQ lies in x + y = 3 plane. Therefore,

P ≡ (α, β, γ)

Q ≡ (0, 0, k)

The midpoint of PQ is (α/2, β/2, (γ + k)/2).

Since the midpoint satisfies x y + = 3, we get

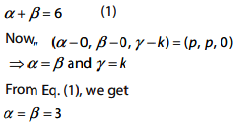
Now, the distance of point P from x-axis is 5. Therefore,