Question

A 2 meter long observer is at 28 meter distance from a tower. The angle of elevation of top of tower from his eyes is 60°. Find the height of tower.

Answer 1

Let AB a tower and CD is a observer. 

The distance between tower and observer = 28 m and angle of elevation is 60° i.e.,

BC = DE = 28 m

CD = BE =2 m and ∠ADE = 60°

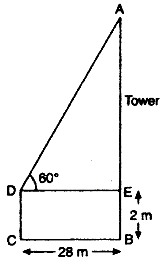
Form right angled ΔAED

tan 60° = AE/ED

⇒ √3 = AE/28

⇒ AB = 28√3 m

= 28 × 1.732 = 48.496 m

Height of tower AB = BE + AE 

= 2 + 48.496

= 50.5 meter (approx)

Hence, height of tower is 50.5 m (approx)