i. The given simultaneous equations are
3x – y – 2 = 0
∴ y = 3x – 2
|
x |
0 |
1 |
3 |
-1 |
|
y |
-2 |
1 |
7 |
-5 |
|
(x,y) |
(0,-2) |
(1,1) |
(3,7) |
(-1,-5) |
2x + y = 8
∴ y = 8 - 2x
|
x |
0 |
4 |
1 |
3 |
|
y |
8 |
0 |
6 |
2 |
|
(x,y) |
(0,8) |
(4,0) |
(1,6) |
(3,2) |
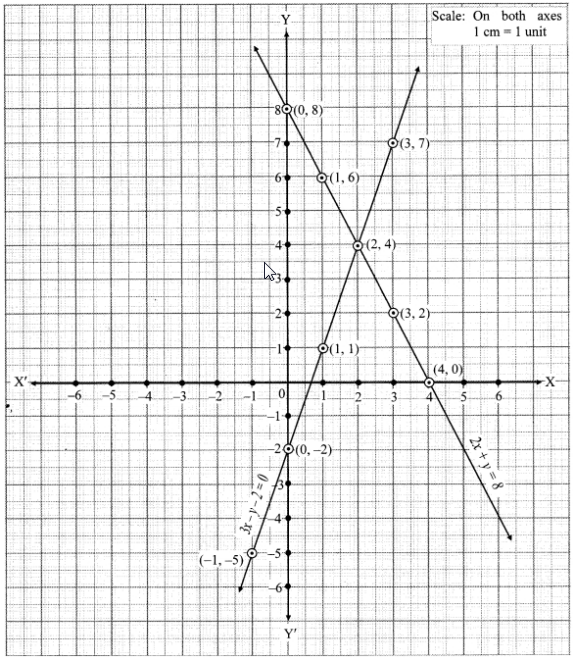
The two lines intersect at point (2, 4).
∴ x = 2 and y = 4 is the solution of the simultaneous equations 3x – y – 2 = 0 and 2x + y = 8.
ii. The given simultaneous equations are
3x + y = 10
∴ y = 10 – 3x
|
x |
2 |
3 |
4 |
5 |
|
y |
4 |
1 |
-2 |
-5 |
|
(x,y) |
(2,4) |
(3,1) |
(4,-2) |
(5,-5) |
x - y = 2
∴ y = x - 2
|
x |
0 |
2 |
4 |
5 |
|
y |
-2 |
0 |
2 |
3 |
|
(x,y) |
(0,-2) |
(2,0) |
(4,2) |
(5,3) |
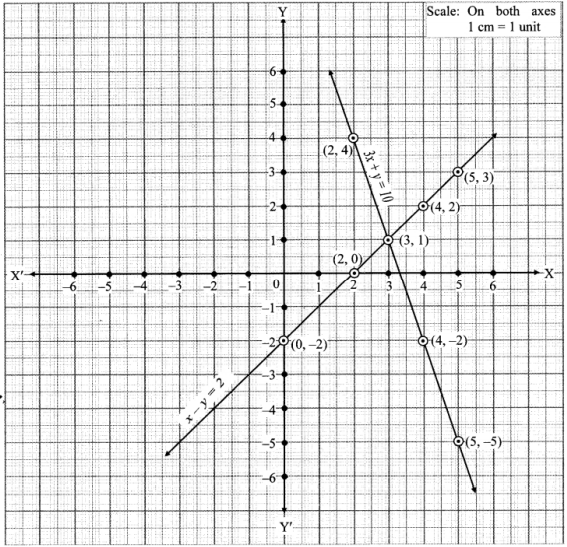
The two lines intersect at point (3, 1).
∴ x = 3 and y = 1 is the solution of the simultaneous equations 3x + y = 10 and x – y = 2