Question

Prove Pythagoras theorem.

Answer 1

Proof of the Pythagoras theorem using similarity of triangles.

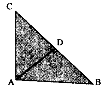
Given: ∠BAC = 90°

To Prove: BC² = AB² + AC²

Construction: Draw AD ⊥ BC

Proof:

In ∆ ABC and ∆ DBA

∠B is common and ∠BAC = ∠ADB = 90°

Therefore, ∆ ABC ~ ∆ DBA, (AA similarity)

Hence, the ratio of corresponding sides are equal.

⇒ ABDB = BCBA

⇒ AB² = BC x DB

Similarity, ∆ ABC ~ ∆ DAC,

⇒ AC/DC = BC/AC

⇒ AC² = BC x DB

Adding (1) and (2), we get

AB² + AC² = BC x DB + BC x DC

= BC x (DB + DC) = BC x BC

⇒ AB² + AC² = BC² and hence the proof of the theorem.