Find the point of the curve y = y = √(x - 3)

Question

Find the point of the curve y = \(\sqrt{x - 3}\) where the tangent is perpendicular to the line 6x + 3y – 5 = 0.

y = √(x - 3)

Answer 1

Let the required point on the curve y = \(\sqrt{x - 3}\) be P(x₁, y₁).

Differentiating y = \(\sqrt{x - 3}\) w.r.t. x, we get

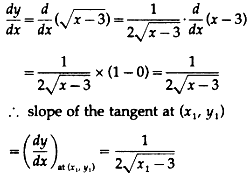
Since, this tangent is perpendicular to 6x + 3y - 5 = 0

whose slope is -6/3 = -2,

slope of the tangent = -1/-2 = 1/2

Hence, the required points are (4, 1) and (4, -1).