Question

Describe the method of measuring angle of repose.

Answer 1

When objects are connected by strings and When objects are connected by strings and a force F is applied either vertically or horizontally or along an inclined plane, it produces a tension T in the string, which affects the acceleration to an extent. Let us discuss various cases for the same.

Case 1: 

Vertical motion: 

Consider two blocks of masses m₁ and m₂ (m₁ > m₂ ) connected by a light and in extensible string that passes over a pulley as shown in Figure.

Let the tension in the string be T and acceleration a. When the system is released, both the blocks start moving, m₂ vertically upward and m_k, downward with same acceleration a. The gravitational force m₁g on mass m₁ is used in lifting the mass m₂. The upward direction is chosen as y direction. The free body diagrams of both masses are shown in Figure.

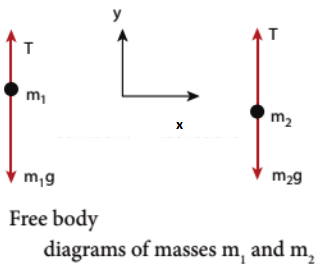
Applying Newton’s second law for mass m₂ T\(\hat j\) – m₂g\(\hat j\) = m₂a\(\hat j\) The left hand side of the above equation is the total force that acts on m₂ and the right hand side is the product of mass and acceleration of m₂ in y direction. 

By comparing the components on both sides, we get

T = m₁g  = m₂a ……….(1)

Similarly, applying Newton’s second law for mass m₂

T\(\hat j\) – m₁ g\(\hat j\) = m₁ a\(\hat j\)

As mass mj moves downward (-\(\hat j\)), its acceleration is along (-\(\hat j\))

By comparing the components on both sides, we get

T = m₁ g = -m₁a 

m₁g – T = m₁a ………..(2)

Adding equations (1) and (2), we get

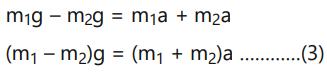
From equation (3), the acceleration of both the masses is –

If both the masses are equal (m₁ = m₂), from equation (4)

a = 0

This shows that if the masses are equal, there is no acceleration and the system as a whole will be at rest.

To find the tension acting on the string, substitute the acceleration from the equation (4) into the equation (1).

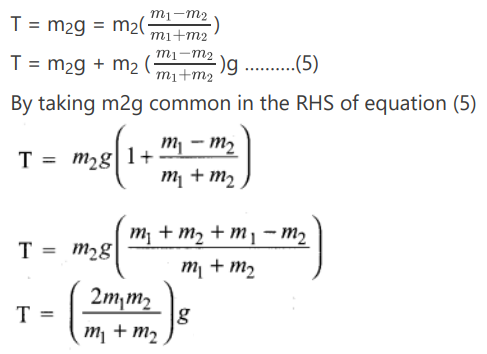
Equation (4) gives only magnitude of acceleration For mass m₁, the acceleration vector is given by \(\vec a\)

= -\(\frac{m_1 - m_2}{m_1 +m_2} \hat j\)

For mass m₂, the acceleration vector is given by \(\vec a\)

= \(\frac{m_1 - m_2}{m_1 +m_2} \hat j\)

Case 2: 

Horizontal motion: 

In this case, mass m₂ is kept on a horizontal table and mass m , is hanging through a small pulley as shown in figure. Assume that there is no friction on the surface

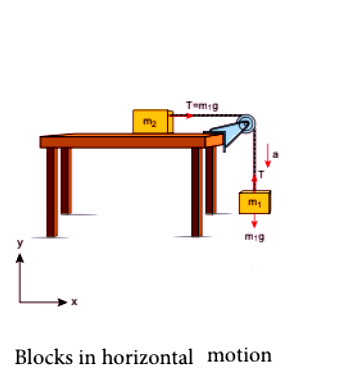
As both the blocks are connected to the unstretchable string, if m₁ moves with an acceleration a downward then m₂ also moves with the same acceleration a horizontally. 

The forces acting on mass m₂ are

1. Downward gravitational force (m₂g) 

2. Upward normal force (N) exerted by the surface 

3. Horizontal tension (T) exerted by the string 

The forces acting on mass m are 

1. Downward gravitational force (m₁g) 

2. Tension (T) acting upwards 

The free body diagrams for both the masses is shown in figure 2.

Applying Newton’s second law for m₁

T\(\hat i\) – m₁g \(\hat j\) = -m₁a\(\hat j\) (alongy direction) 

By comparing the components on both sides of the above equation, 

T – m₁g = -m₁a …………(1) 

Applying Newton’s second law for m₂ 

T\(\hat i\) = m₁a\(\hat i\) (along x direction) 

By comparing the components on both sides of above equation,

T = m₂a ………….(2)

There is no acceleration along y direction for m₂ .

N\(\hat j\) – m₂g\(\hat j\) = 0

By comparing the components on both sides of the above equation

N – m₂ g = 0 

N = m₂ g ……….(3) 

By substituting equation (2) in equation (1), we can find the tension T

m₂a – m₁g = -m₁ a

m₂a + m₁a = m₁g

a = \(\frac{m_1}{m_1 + m_2}\) g …………(4)

Tension in the string can be obtained by substituting equation (4) in equation (2)

T = \(\frac{m_1 m_2}{m_1 + m_2}\)g ………..(5)

Comparing motion in both cases, it is clear that the tension in the string for horizontal motion is half of the tension for vertical motion for same set of masses and strings. This result has an important application in industries. The ropes used in conveyor belts (horizontal motion) work for longer duration than those of cranes and lifts (vertical motion).