Question

The initial rate of reactions

3A + 2B + C → products at different initial concentrations are given below.

Initial rate ms-1	[A0] M	[A0] M	[C0] M
1) 5.0 x 10-3	0.010	0.005	0.010
2) 5.0 x 10-3	0.010	0.005	0.015
3) 1.0 x 10-2	0.010	0.010	0.010
4) 1.25 x 10-3	0.005	0.005	0.010

Answer 1

(i) Consider equation (1) and (2),

The concentration of A and B are constant. The rate remains the same for different concentrations of C. Hence, the order with respect to C is zero.

(ii) Consider equations (3) and (1),

The concentration of A and C are constant. The concentration of B is doubled, rate increases by 2 times. Hence order with respect to B is 1.

(iii) Consider equations (4) and (1),

The concentration of B and C remain constant. When the concentration of A is doubled, the rate increases by 4 times. Hence order with respect to A is 2.

Alternate method:

Suppose order with respect to A, B and C are α, β and γ respectively. Then the rate law at different concentration of A, B and C are

5.0 × 10^-3 = k [0.010]^α [0.005]^β [0.010]^γ …(1)

5.0 × 10^-3 = k [0.010]^α [0.005]^β [0.015]^γ …(2)

1.0 × 10^-3 = k [0.010]^α [0.010]^β [0.010]^γ …(3)

1.25 × 10^-3 = k [0.005]^α [0.005]^β [0.010]^γ …(4)

Dividing (1) by (2),

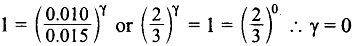
i.e., order with respect to C is zero.

Dividing (3) by (2),

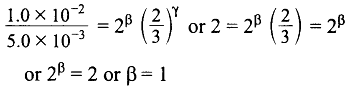
Dividing (1) by (4),

⇒ 4 = 2^α or α = 2

Order with respect to A, B, C are 2, 1 and 0.